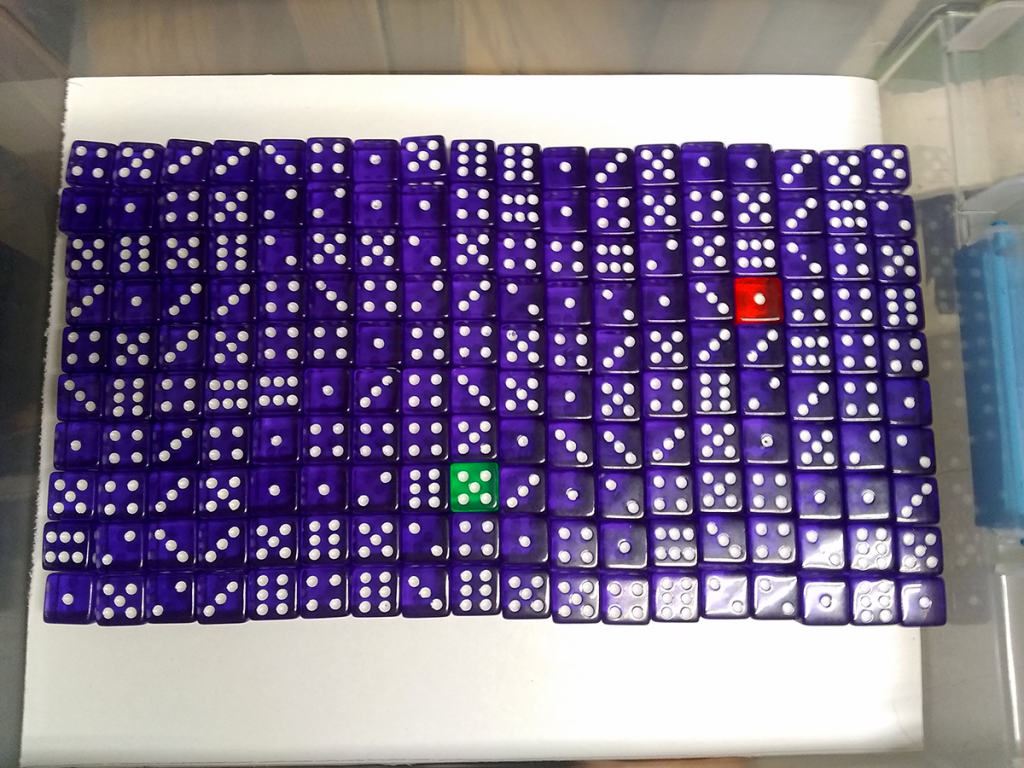
Dice! Bins of colorful dice, each with 178 of one bold color, plus two going their own way. Each bin arrayed in a 10 x 18 or 12 x 15 grid, per the shop tech’s preference at that moment. Beats counting them one by one.
Secure the lid and shake with all your might: you’re simulating radioactive decay! Loudly.
Pick a number from one to six. Say, three. Each die that turns up with three pips after a shake decays, and you remove it from the bin. With 180 dice in there, the chances of getting all threes – or zero threes – is vanishingly small. One-in-six raised to the 180th power, right? As a percentage that’s, what, nearly 140 zeros after the decimal point? Run the numbers, and you can look forward to around one-sixth of the dice in there decaying with each shake. Sometimes more, sometimes less.
You’ll also keep a close eye on those differently-colored dice. One for you, one for your partner. They’re the atoms you’re watching carefully, and unlike the sorta-predictable rolls of a large mass of dice, they’ll decay when they’re good and ready. Could be first, could be never. It’s an illustration of how probability works in systems of different sizes. Of how the random nature of radioactive decay produces a predictability with enough atoms and enough time.
In some idealized version of this experiment, you’d have 30 dice decay on the first shake. Then 25. Then 21. 17. 15. 12. 10. 8. 7. 6. 5. 4. 3. 3. 2. 2. 2. 1. 1. 1. After that… maybe one per shake? (The student experiment stops well before you’re down to a meager handful of dice.) The half-life arrives around four shakes. Every four shakes. Neat!
And should the effect with 180 dice not be enough? Compare your data to the rest of the lab, seeing how each rate of decay is nearly but not exactly the same. Then aggregate the data from all dozen lab benches. 2,160 dice decaying.
Loudly.